SOLUTION
Given the question in the image, the following are the solution steps to answer the question.
STEP 1: Write the given expression
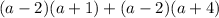
STEP 2: Separate the expression into 2 parts
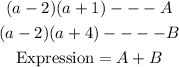
STEP 3: Factorize Part A
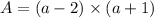
STEP 4: Factorize Part B
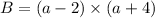
STEP 5: Write out the common factor
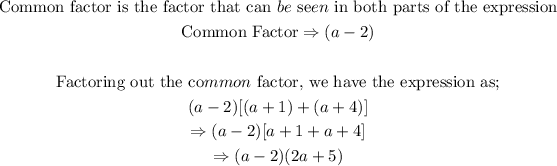
Hence, the correct choice is A and the answer in factored form is:
