Question

The graph of y = ax + bx + c is a parabola that opens up and has a vertex at (0,5). What is the solution set of the related equation 0 = ax2 + bx + c? © O{0] (5) NEXT QUESTION TURN IT IN ASK FOR HELP i LE

Answer 1

Vertex form of a parabola: y= a(x-h)^2 + k

vertex = (h,k) = (0,5)

Replacing:

y= a(x)^2 + 5

We have to solve for:

0 = ax^2 + bx + c

a = a

b= 0

c = 5

Apply the quadratic formula:

`(-b\pm√(b^2-4* a* c))/(2* a)`

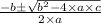
Replacing:

`(0\pm√(0-4* a*5))/(2* a)`
`(√(-20a))/(2a)`

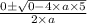
a must be negatve

Since there is a negative number under the root there is no real solution.

Answer: ∅