Question

Look at the following sequence:1/3 3/12 9/48 27/192If it is a geometric sequence, choose the common ratio. If it is not a geometric sequence, choose "not geometric."

Answer 1

For finding if its a geometric sequence, we will do the quotient between a value of the sequence and the one before it. If we obtain the same number, this will be the common ratio.

For the terms 1 and 2:

`((3)/(12))/((1)/(3))=(9)/(12)=(3)/(4)=0.75`

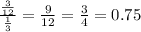
For the terms 2 and 3:

`((9)/(48))/((3)/(12))=(9\cdot12)/(3\cdot48)=(108)/(144)=(54)/(72)=(27)/(36)=(9)/(12)=(3)/(4)=0.75`

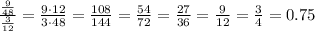
For the terms 3 and 4:

`((27)/(192))/((9)/(48))=(27\cdot48)/(192\cdot9)=(3\cdot48)/(192)=(3\cdot24)/(96)=(24)/(32)=(12)/(18)=(3)/(4)=0.75`

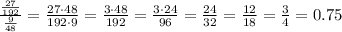
Finally, as all quotients give the same number, we conclude that the initial sequence is a geometric one, and that its common ratio is 0.75.