give data:
The currve moving along with point P is,
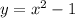
The line moving along the point Q is,
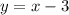
Let us now graph the given curve and line,
The closest point for the curve moving point P is,
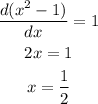
thus,
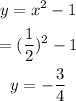
The distance between the two given paths is the perpendicular distance from the line y=x-3 to the point (1/2,-3/4).
The equation of the line in point and slope form is,

at the coordinates of point Q is the distance between the two points have minimum value.,
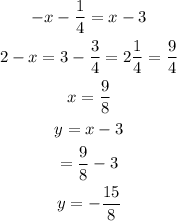
The point is (9/8,-15/8)
now we find the distance between the point by using the formula,'
![\begin{gathered} \text{length}=\sqrt[]{(y_2-y_1)^2+(^{_{}}x}_2-x_1)^2 \\ \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/z8lpgn7sjwj48h6x8knd.png)
thus, the distance is,
![\begin{gathered} \sqrt[]{((9)/(8)-(1)/(2))^2+(-(15)/(8)-(-(3)/(4)))^2} \\ =\sqrt[]{(53)/(32)} \\ length=1.28 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/6742cn6n0637dn9h3z8l.png)