Question

Which of the following is a solution of x² + 14x + 112 = 0? If necessary, round to the nearest hundredth.

x = –0.24
x = –4.24
x = 4.24
There is no solution.

Answer 1

The given equation has no solution.

Explanation:

Given the equation
`x^2+14x+112=0`

we have to find the solution of the above equation.

The roots of quadratic equation
`ax^2+bx+c=0` is

`x=(-b\pm √(b^2-4ac))/(2a)`

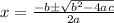
Equation :
`x^2+14x+112=0`

The roots are

`x=(-14\pm √(14^2-4(1)(112)))/(2)`

`x=(-14\pm √(196-448))/(2)=(-14\pm √(-252))/(2)`

Since, determinant under the root is negative

⇒ The given equation has no real solution.

Last option is correct.

Answer 2

To determine the solution of the quadratic equation, use the quadratic formula which states that,
x = ((-b +/- sqrt (b² - 4ac)) / 2a
From the equation, a = 1, b = 14, and c = 112. Substituting these to the quadratic formula,
x = ((-14 +/- sqrt (14² - 4(1)(12)) / 2(1) = indeterminate
Thus, the equation does not a real number solution.