Question

Find the slope of the line through the points (4, -1) and (1, -4) and then graph it. Slope=

Answer 1

Given:

Line pass through the point ( 4, -1 ) and ( 1, -4 )

Find-:

The slope of the line and graph of the line.

Explanation-:

The slope of the line is:

`m=(y_2-y_1)/(x_2-x_1)`

Where,

`\begin{gathered} m=\text{ Slope} \\ \\ (x_1,y_1)=\text{ First point} \\ \\ (x_2,y_2)=\text{ Second point} \end{gathered}`

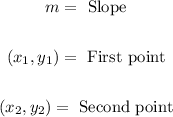
Given point is:

`\begin{gathered} (x_1,y_1)=(4,-1) \\ \\ (x_2,y_2)=(1,-4) \end{gathered}`

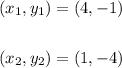
The slope of the line is:

`\begin{gathered} m=(y_2-y_1)/(x_2-x_1) \\ \\ m=(-4-(-1))/(1-4) \\ \\ m=(-4+1)/(1-4) \\ \\ m=(-3)/(-3) \\ \\ m=1 \end{gathered}`

The slope of the line is 1.

For a graph of lines,

Equation of line is:

`y=mx+c`

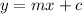
Where,

`\begin{gathered} m=\text{ Slope} \\ \\ c=Y-\text{ intercept} \end{gathered}`

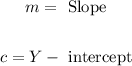
So, the equation become

`\begin{gathered} y=mx+c \\ \\ y=1x+c \\ \\ y=x+c \end{gathered}`

For value of "c" is:

Point = ( 4, -1)

`\begin{gathered} y=x+c \\ \\ (x,y)=(4,-1) \\ \\ y=x+c \\ \\ -1=4+c \\ \\ c=-1-4 \\ \\ c=-5 \end{gathered}`

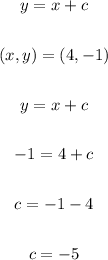
So, the equation

`\begin{gathered} y=mx+c \\ \\ y=x-5 \end{gathered}`

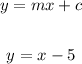
So, the graph of line is: