Question

The admission fee at an amusement park is $4.00 for children and $5.80 for adults. On a certain day, 296 people entered the park, and the admission fees collected totaled 1472 dollars. How many children and how many adults were admitted?

Answer 1

Given;

Admission fee for children is, f(C) = $4.

Admission fee for adult is, f(A) = $5.80.

Total number of people is, N = 296.

Total fees collected is, F = $1472.

The objective is to find the number of children and number of adults.

Consider the childrens as C and the adults as A.

Then, first population equation can be written as,

`C+A=296`

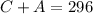
Then, first cost equation can be written as,

`4C+5.8A=1472`

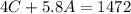
Solve the two equations by multiplying the first equation by 4.

`\begin{gathered} 4C+4A=1184 \\ 4C+5.8A=1472 \end{gathered}`

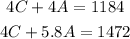
On solving,

`\begin{gathered} -1.8A=-288 \\ A=(288)/(1.8) \\ A=160 \end{gathered}`

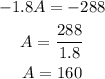
Substitute the value of A in equation (1) to finf the value of C.

`\begin{gathered} C+160=296 \\ C=296-160 \\ C=136 \end{gathered}`

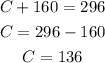
Hence, the number of children is 136 and the number of adult is 160.