Question

BC has one endpointB(3,2) and a midpointT(6,-2). Find thecoordinates of theother endpoint, C. Thank you in advance!!

Answer 1

We are given that BC has one endpoint B(3, 2) and a midpoint T(6, -2)

We are asked to find the coordinates of the other endpoint C.

Recall that the midpoint formula is given by

`T(x,y)=((x_1+x_2)/(2),(y_1+y_2)/(2))`

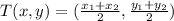
Where

`\begin{gathered} T(x,y)=(6,-2) \\ B(x_1,y_1)=(3,2) \end{gathered}`

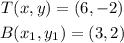
So, the other endpoint C is

`x=(x_1+x_2)/(2),y=(y_1+y_2)/(2)`

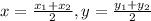
Substitute the given values,

`6=(3+x_2)/(2),-2=(2+y_2)/(2)`

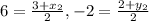
Simplify,

`\begin{gathered} 2\cdot6=3+x_2,-2\cdot2=2+y_2 \\ 12=3+x_2,-4=2+y_2 \\ x_2=12-3,y_2=-4-2 \\ x_2=9,y_2=-6 \end{gathered}`

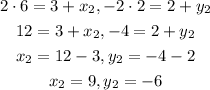
Therefore, the coordinates of the endpoint C are

`C(x_2,y_2)=(9,-6)`

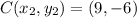
Bonus:

Let us verify whether we got the correct coordinates or not.

`\begin{gathered} T(x,y)=((x_1+x_2)/(2),(y_1+y_2)/(2)) \\ T(x,y)=((3+9)/(2),(2-6)/(2)) \\ T(x,y)=((12)/(2),(-4)/(2)) \\ T(x,y)=(6,-2) \end{gathered}`

Hence, we got the same midpoint as given in the question.