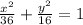From the given picture, we can to note that the major axis is located on the x-axis. So, the ellipse equation has the form
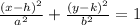
where (h,k) is the coordinate of the center, a is the distance from the center one vertex (the one located on the major axis) and b is the distance from the center to the minor axis vertex, that is,
Then, from the picture above, we can note that
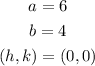
So, by substituting these values into our first equation, we get
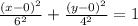
Therefore, the answer the ellipse equation is: