Question

A body was found at 10 a.m. outdoors on a day when the temperature was 40oF. The medical examiner found the temperature of the body to be 80oF. What was the approximate time of death? Use Newton's law of cooling, with k = 0.1947.

Answer 1

8 am

Step-by-step explanation:

Equation of newtons law of cooling is -

`T(t) = T_(A) + (T_(o) - T_(A) ) e^(-kt)`

Where
`T_(o)` is ambient temperature which is equal to
`98.6`

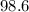
`T(t) =` 80 degree Fahrenheit

`T_(A) = 40`degree Fahrenheit

`k = 0.1947`

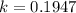
Substituting the given values in above equation we get -

`80 = 40 + (98.6 - 40) e^(-0.1947t) \\40 = 58.6 e^(-0.1947t)\\(40)/(58.6)  = e^(-0.1947t) \\`

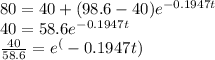
Taking log on both sides we get -

`ln(40)/(58.6)  = -0.1947t \\-0.38 = -0.1947t\\t = 1.95`hour

which is almost 2 hour

So the time of death would be two hour before 10 am

i.e 8 am

Answer 2

We are given with the data that at 10 am, the temp is 40oF. We are asked for the time when the body's temperature is equal to 80oF. In this casem we use Newtons law of cooling: T (t) = Ta + (To - Ta) e -kt. Substituing, 98.6 = 40 + (80-40) e -0.1947t. t is equal to . Hence the approximate time of death is -1.96 hrs ago or approx two hours ago. The answer is 8 am.