Question

If HI is not parallel to FG, what is mZG?1350HGFmZG=

Answer 1

The sides IF and HG are parallel, so we have a trapezoid IHGF.

Since the sides IH and FG have the same length, we have an isosceles trapezoid.

That means the angles ∠I and ∠F are congruent, so the angle ∠F is equal 35°.

Since the figure is a trapezoid, the angles ∠F and ∠G are supplementary (because they are consecutive interior angles), so we have:

`\begin{gathered} \angle F+\angle G=180 \\ 35+\angle G=180 \\ \angle G=180-35 \\ \angle G=145\degree \end{gathered}`

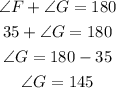
So the angle ∠G is equal to 145°.