Question

Find the mean, standard deviation, and five-number summary for the data set. Assume population data are given. 9, 15, 15, 21, 23, 31, 33, 37, 45, 51

Answer 1

Let's find the mean.

`\begin{gathered} \bar{x}=(\Sigma(x))/(N)=(9+15+15+21+23+31+33+37+45+51)/(10) \\ \bar{x}=(280)/(10)=28 \end{gathered}`

The mean is 28.

Then, we find the standard deviation

`\sigma=\sqrt[]{\frac{\Sigma(x-\bar{x})^2}{N}}`

Let's find the difference between each value and the mean

9-28 = -19

15-28=-13

15-28=-13

21-28=-7

23-28=-5

31-28=3

33-28=5

37-28=9

45-28=17

51-28=23

Then, we add the square power of each subtraction

`\begin{gathered} \sigma=\sqrt[]{((-19)^2+(-13)^2+(-13)^2+(-7)^2+(-5)^2+3^2+5^2+9^2+17^2+23^2)/(10)} \\ \sigma=\sqrt[]{(1706)/(10)} \\ \sigma\approx13.1 \end{gathered}`

The standard deviation is 13.1.

On the other hand, the five-number summary refers to the minimum, the first quartile, the median, the third quartile, and the maximum.

According to the given data set, we have

• The minimum is 9.

,

• The maximum is 51.

,

• The first quartile is 15. (the middle value between the first 5 numbers)

,

• The third quartile is 37. (the middle value between the second 5 numbers)

,

• The median is between 23 and 31.

`Me=(23+31)/(2)=(54)/(2)=27`

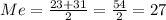
• The median is 27.