Question

F(x)= x^3 +3G(x)= x^2 +2Approximate the solution to the equation f(x) = g(x) using three iterations of successive approximation. Use this graph as a starting point.

Answer 1

`f(x)=x^3+\text{ 3}`

`g(x)=x^2\text{ + 2}`

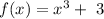
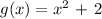
At the point of intersection, f(x)=g(x)

`\begin{gathered} x^3+3=x^2\text{ + 2} \\ \text{collect like terms} \\ x^3-x^2\text{ +3 - 2 = 0} \\ x^{3\text{ }}-x^2+\text{ 1= 0} \end{gathered}`

`x^3-x^2+1=\text{ 0}`

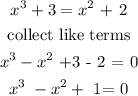
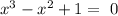
The above graph is that of the equation

`x^3-x^2\text{ + 1 = 0}`

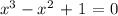
The solution is = -0.755 which is approximately -0.8

From the options provided

For option A, x= -13/16 = -0.8125

For option B, x = -5/4 = -1.25

For option C, x = -15/16 = -0.9375

For option D, x = -7/8 = -0.875

From the options provided, The closest to the solution is Option A

Because - 0.8125 is approximately -0.8