Let
 be the number of loaves of bread that Helena can bake, and let
be the number of loaves of bread that Helena can bake, and let
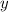 be the number of batches muffins she can bake.
be the number of batches muffins she can bake.
We know from our problem that she needs 3.5 cups of flour per loaf of bread and 2.5 cups of flour per batch of muffins. Since
 is the number of loaves of bread and
is the number of loaves of bread and
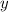 is the number of muffins, we can express this as
is the number of muffins, we can express this as
 . We also now that she has 17 cups of flour, so the cups of flour in the breads and muffins must be equal to 17; in other words:
. We also now that she has 17 cups of flour, so the cups of flour in the breads and muffins must be equal to 17; in other words:
 This is going to be equation (1)
This is going to be equation (1)
We also know from our problem that she needs 0.75 cup of sugar per loaf of bread and 0.75 cup of sugar per batch of muffins. Since
 is the number of loaves of bread and
is the number of loaves of bread and
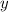 is the number of batches of muffins, we can express this as
is the number of batches of muffins, we can express this as
 . We also know that she has a total of 4.5 cups of sugar available for baking, so the total sugar in brads and muffins must be 4.5 cups; in other words:
. We also know that she has a total of 4.5 cups of sugar available for baking, so the total sugar in brads and muffins must be 4.5 cups; in other words:
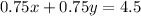 This is going to be equation (2)
This is going to be equation (2)
We created a system of equations with the information in our problem, so let's solve the system to find how much of each she can bake.
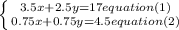
Step 1. Solve for
 in equation (2)
in equation (2)
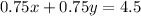
![0.75x=4.5-0.75y [/tex</p><p>[tex] x=(4.5-0.75y)/(0.75)](https://img.qammunity.org/2017/formulas/mathematics/high-school/bl4czuc68vtq8cd2swnkj3oaymq7tmwbv9.png)
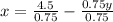
 equation (3)
equation (3)
Step 2. Replace equation (3) in equation (1) and solve for
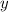
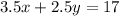
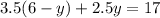
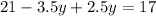

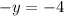
 equation (4)
equation (4)
Step 3. Replace equation (4) in equation (3)


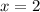
We can conclude that Helena can bake 2 loaves of bread and 4 batches of muffins with 17 cups of four and 4.5 cups of sugar.