Question

Given the geometric sequence where a1 = 3 and the common ratio is -1, what is the domain for n? a. All integers where ≤ 0. b. All integers where n ≥ 0. c. All integers where n ≤ 1. d. All integers where n ≥ 1 . . Would the answer be A considering that the ration is a negative?

Answer 1

all integers where n > 1

Explanation:

Answer 2

Option: d is the correct answer.

The domain for n is all the integers where n≥1.

Explanation:

We know that a geometric sequence also known as geometric progression in which each term is found by multiplying the preceding term by a constant factor.

That common factor is also known as a common ratio and is denoted by 'r'.

Now, the sequence is given as:

`a_1=a\\\\a_2=ar\\\\a_3=ar^2\\\\a_4=ar^3\\.\\.\\.\\.\\.\\.\\.\\.\\.`

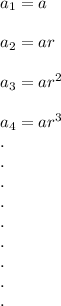
Hence, the nth term of the sequence is given by:

`a_n` where n belongs to natural numbers.

Where a is the first term of the sequence i.e. when n=1

Hence, the domain for n is:

d. All integers where n ≥ 1