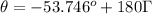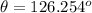Vectors
Given a vector of components A = (Ax, Ay), the magnitude of the vector is:
![|A|=\sqrt[]{A^2_x+A^2_y}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/vz8dq42tna52plr7fiy0.png)
And the angle in standard form (counterclockwise from the positive x-direction is given by:
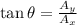
The vector A has components Ax = -5.50 m and Ay = 7.50 m. The magnitude is calculated as follows:
![\begin{gathered} |A|=\sqrt[]{(-5,50)^2+7.50^2} \\ \text{Operating:} \\ |A|=\sqrt[]{30.25+56.25} \\ |A|=\sqrt[]{86.5} \\ |A|=9.3 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/lppe3o1gmjmwsc8p0qo4.png)
The magnitude of A is 9.3 m.
Calculate the angle. It's important to notice the x-coordinate is negative and the y-coordinate is positive, thus the angle is in quadrant II (between 90° and 180°).
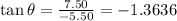
Calculating the angle with the inverse tangent function:
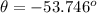
This angle is not expressed correctly in the second quadrant, so we have to add 180°: