Question

The pressure of 1 mol of gas is decreased to 0.5 atm at 273 K. What happens to the molar volume of the gas under these conditions?

Answer 1

The molar volume of a gas at STP is the volume of one mole of the gas at 0°C (273.15 K) and 1 atm pressure. If the pressure of 1 mol of gas is decreased to 0.5 atm at 273 K, the molar volume of the gas would increase.

Step-by-step explanation:

The molar volume of a gas is the volume of one mole of a gas at standard temperature and pressure (STP), which is 0°C (273.15 K) and 1 atm pressure. According to the ideal gas law, PV = nRT, where P is the pressure, V is the volume, n is the number of moles, R is the ideal gas constant, and T is the temperature in Kelvin. If the pressure of 1 mol of gas is decreased to 0.5 atm at 273 K, the molar volume of the gas would increase because the pressure is inversely proportional to the volume.

Answer 2

Answer : The molar volume of the gas will be, 44.82 L

Solution :

Using ideal gas equation,

`PV=nRT`

where,

n = number of moles of gas = 1 mole

P = pressure of the gas = 0.5 atm

T = temperature of the gas = 273 K

R = gas constant = 0.0821 Latm/moleK

V = volume of the gas.

Now put all the given values in the above equation, we get the molar volume of the gas.

`(0.5atm)* V=(1mole)* (0.0821Latm/moleK)* (273K)`

`V=44.82L`

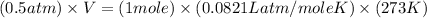
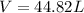
Therefore, the molar volume of the gas will be, 44.82 L