Question

The equation of a circle is (x - 3)2 + (y + 2)2 = 25. The point (8, -2) is on the circle.

What is the equation of the line that is tangent to the circle at (8, -2)?

y = 8
x = 8
x = 3
y = 3

Answer 1

Hello,

Answer A

y=8
=======================

Answer 2

2nd Option is correct.

Explanation:

Given Equation of Circle,

( x - 3 )² + ( y + 2 )² = 25

Point on circle = ( 8 , -2 )

To find: The equation of the line that is tangent to the circle at given point.

By comparing equation of circle with standard equation of circle,

( x - h )² + ( y - k )² = r²

we get,

Coordinates of center = ( 3 , -2 )

Slope of the radius line from center to point ( 8 , -2 ) =
`(-2-(-2))/(8-3)=0`

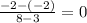
Since, y-coordinate of radius line is same.

⇒ This line is parallel to x-axis.

We also knows that Radius and tangent are perpendicular to each other at point of contact.

⇒ Tangent is Parallel to y-axis and passes through ( 8 , -2 )

⇒ Equation of Tangent is x = 8

Therefore, 2nd Option is correct.