Question

What are the vertex, focus, and directrix of the parabola with the given equation?

12y = x^2 –6x + 45 (1 point)

Answer 1

y=(((x^2)-(6*x)+45)/12)

Answer 2

Vertex is (3,3)

Focus (3,6)

Directrix y=0

Explanation:

We are given that an equation of parabola

`12y=x^2-6x+45`

Subtract 45 on bot sides then we get

`12y-45=x^2-6x`

To make complete square on right side

We add 9 on both sides

`12y-45+9=x^2-6y+9`

`12y-36=(x-3)^2` (
`(a-b)^2=a^2+b^2-2ab,(x-3)^2=x^2-6x+9)`

`12(y-3)=(x-3)^2`

Compare it with the equation of parabola along y- axis

`(x-h)^2=4p(y-k)`

Then, we get h=3,k=
`3`

`4p=12`

`p=(12)/(4)=3`

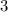
Vertex of parabola =(h,k)=(3,3)

Focus of parabola=(x,p+k)=(3,3+3)=(3,6)

Equation of directrix of parabola ,y=k-p=3-3=0