Question

A triangle has vertices P(1, 3), Q(3, 5), and R(6, 2).

The slope of the median to QR is
a. 1/7
b. -1/2
c. 1
Can you lead me through the steps cuz i don't get how to do...?

Answer 1

Hello,

M, middle of [QR] has as coordinate (9/2,7/2)

The slope of the line PM is (3-7/2)/(1-9/2)=(-1/2) / (-7/2)=1/7.

Just as you found !

Answer 2

The slope of the median to QR is 1/7

Explanation:

A triangle has vertices P(1, 3), Q(3, 5), and R(6, 2).

To find the slope of the median to QR is

Mid point of QR is
`((x1+x2)/(2) , (y1+y2)/(2))`

Q(3, 5), and R(6, 2)

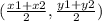
`((3+6)/(2) , (5+2)/(2))`

`((9)/(2) , (7)/(2))`

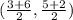
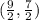
Now we find the slope using mid point (9/2, 7/2) and vertex (1,3)

slope =
`(y2-y1)/(x2-x1) =(3-(7)/(2))/(1-(9)/(2)) =(1)/(7)`