Question

Hello, I need help with this 8th Grade Mathematics question.

Answer 1

We have to find the equation of the line.

To find the equation, we only need two points that belong to the line.

We can pick the points (0,-1) and (6,3) and calculate the slope as:

`m=(y_2-y_1)/(x_2-x_1)=(3-(-1))/(6-0)=(4)/(6)=(2)/(3)`

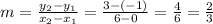
The point (0,-1) gives us the y-intercept b = -1, so we can write the equation of the line as:

`y=(2)/(3)x-1`

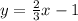
We have to find the coordinates for the point (a,b).

We have infinite possibilities for a, and then b is found using the equation of the line.

We can see that a, the x-coordinate, correspond to x = 3, so a = 3.

Then, we can calculate b using the equation of the line:

`b=y(a)=y(3)=(2)/(3)(3)-1=2-1=1`

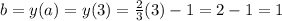
Then, we have (a,b) = (3,1).

The y-coordinate when x = 0 is y = -1, as we know the point (0, -1) that belongs to the line.

Answer:

The slope of the line is m = 2/3.

The equation of the line is y = 2/3*x -1

The values of a and b are a = 3 and b = 1.

When x = 0, the y-coordinate is y = -1.