Question

a rectangular auditorium seats 1075 people. the number of seats in each row exceeds the number of rows by 18. find the number of seats in each row

Answer 1

Given:

The total number of seat is 1075.

The number of seats in each row exceeds the number of rows by 18.

The objective is to find the number of seat in each row.

Consider the number of rows as x.

If the number of seats in each row exceeds the number of row by 18, then the total seat can be represented as,

`\begin{gathered} x\cdot(x+18)=1075 \\ x^2+18x=1075 \\ x^2+18x-1075=0 \end{gathered}`

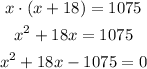
Consider a = 1, b = 18 and c = -1075.

On factorizing the above quadratic equation using quadratic formula,

`\begin{gathered} x=\frac{-b\pm\text{ }\sqrt[]{b^2-4ac}}{2a} \\ x=\frac{-18\pm\text{ }\sqrt[]{18^2-4(1)(-1075)}}{2(1)_{}} \\ x=\frac{-18\pm\text{ }\sqrt[]{324+4300}}{2_{}} \\ x=\frac{-18\pm\text{ }\sqrt[]{4624}}{2_{}} \\ x=\frac{-18\pm\text{ }68}{2_{}} \\ x=\frac{-18+\text{ }68}{2_{}},\frac{-18-\text{ }68}{2_{}} \\ x=25,-43 \end{gathered}`

Since, negative 43 is not possible, the only possible answer is 25.

Then, the number of seats in a row will be,

`\begin{gathered} \text{Seats}=x+18 \\ =25+18 \\ =43 \end{gathered}`

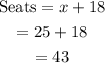
Hence, the total number of seats in a row is 43.