You have the following information:
- Blake and Scarlett spent the same amount of time in reading an entire novel.
- There were 16 chapters in Blake's novel
- There were 25 chapters in Scarlett's novel
- Blake read 6 chapters on first day
- Scarlett read 10 chapters on first day
- Scarlett read 3 chapters per hour on second day
If Scarlett read 10 chapters on first day, then, she read the last 15 chapter on second day (because the novel has 25 chapters).
If Scarlett read 3 chapter per hour on second day, she read the last 15 chapters in the following time:
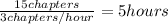
Thus, Scarlett read the last 15 chapters on 5 hours.
If Blake read 16 chapters on first day, it is necessary that he read 10 chapters on the second day. Due to both Blake and Scarlett spent the same time reading their novels, it is necessary that Blake read the last 10 chapters on 5 hours.
The time Blake spent ending the novel on second day can be written as 10x, where x is the chapters per time he read. Furthermore, time Blakes spent reading the nolvel (10x) is the same time Scarlett spent reading hers (5 hours). Thus you have:
10x = 5
Next, you solve the previoues equation for x:
10x = 5 divide by 10 both sides
x = 5/10
x = 1/2
Hence, Blakes read one half chaper per hour on second day