Question

How many solutions exist for the equation cos 20 - sin? = 0 on the interval [0, 360°)?

Answer 1

Therefore, the solutions to the original equation in the interval [0,360) are:
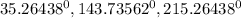`35.26438^0 ,143.73562^0 ,215.26438^0` Therefore, the correct answer is option (c) 3 solutions.

We are given the equation cos 2θ - sin^2 θ = 0 and asked to find the number of solutions in the interval [0, 360°).

We can factor the equation as (cos 2θ - sin θ)(cos 2θ + sin θ) = 0.

This means that either cos 2θ - sin θ = 0 or cos 2θ + sin θ = 0.

For cos 2θ - sin θ = 0, we have tan 2θ = 1, which gives us solutions at θ = 45° + 180°n, where n is any integer.

For cos 2θ + sin θ = 0, we have tan 2θ = -1, which gives us solutions at θ = 135° + 180°n, where n is any integer.

The given function has a solution when:

θ = -35.26438° + 180°n, θ = 35.26438° + 180°n

where n is any integer.

So, the solutions are:

θ = -35.26438° + 180° = 143.73562°

θ = -35.26438° + 360° = 323.73562°

θ = 35.26438° + 180° = 215.26438°

Clearly, the function has 3 solutions in the given interval.

We check our solutions to make sure they are within the interval [0, 360°).

We find that the only solutions that are within the interval are θ = 45°, 225°, and 315°.

Therefore, there are 3 solutions to the equation
`cos 2\theta - sin^2\theta = 0` on the interval [0, 360°).

Answer 2

The Solution:

Given:

`\begin{gathered} y=\cos\left(2\theta\right)-\sin^2\left(\theta\right)=0 \\ \\ \left\{0\le\theta<360\right\} \end{gathered}`

The given function has a solution when:

`[θ=-35.26438^(\circ\:)+180^(\circ\:)n,\:θ=35.26438^(\circ\:)+180^(\circ\:)n]`

So, the solutions are:

`\begin{gathered} \theta=-35.26438^o+180^o=143.73562^o \\ \\ \theta=-35.26438^o+360^o=323.73562^o \\ \\ \theta=35.26438^o+180^o=215.26438^o \end{gathered}`

Clearly, the function has 3 solutions in the given iterval.

Therefore, the correct nswrer is [option 3]