Question

F(x) = log4 (x+10)-2 i need help with the x intercept and end behavior

Answer 1

The function is:

`F(x)=\log _4(x+10)-2`

To find the x-intercept, we let y = 0. Here, y is the function, F(x), so we have:

`0=\log _4(x+10)-2`

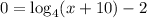
We need to solve this for x, the x-intercept. Shown below:

`\begin{gathered} 0=\log _4(x+10)-2 \\ \log _4(x+10)=2 \\ x+10=4^2 \\ x+10=16 \\ x=16-10 \\ x=6 \end{gathered}`

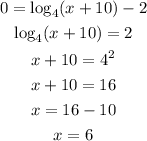
Hence,

x-intercept (6,0)

The end behavior is how the function behaves, or what it approaches, when x goes to infinity or negative infinity.

Let's look at its graph:

As u can see from the graph, the end-behavior:

• as x approaches negative infinity, y goes to negative infinity

,

• as x approaches infinity, y goes to infinity