Question

The width of a rectangular garden is 11 feet longer than 3 times its length. If the garden's perimeter is150 feet, what are the dimensions of the garden?Length =feetWidth =feet

Answer 1

Let the length of the garden be x and the width be y.

It is given that the width of a rectangular garden is 11 feet longer than 3 times its length so it follows:

`\begin{gathered} y=11+3x \\ 3x-y=-11\ldots(i) \end{gathered}`

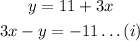
It is also given that the garden's perimeter is 150 feet so it follows:

`\begin{gathered} 2(x+y)=150 \\ x+y=75\ldots(ii) \end{gathered}`

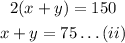
Add equation (i) and (ii) to get:

`\begin{gathered} 3x-y=-11 \\ + \\ x+y=75 \\ 4x=64 \\ x=16 \end{gathered}`

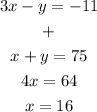
Substitute x=16 in (ii) to get:

`\begin{gathered} 16+y=75 \\ y=59 \end{gathered}`

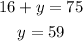
Therefore the dimensions are:

Length=16 feet

Width=59 feet.