Step-by-step explanation
In this problem, we have to:
• a) determine the number of intersections between two functions,
,
• b) find the points of intersection.
The discriminant of a polynomial y = ax² + bx + c is given by:
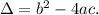
According to the value of the discriminant, we have:
• for Δ > 0 ⇒ two solutions,
,
• for Δ = 0 ⇒ one solution,
,
• for Δ < 0 ⇒ no solutions.
The solutions for y = 0 are given by:
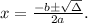
ii) We have the functions:

(a) At the intersection of the functions, we have:
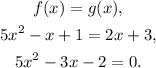
Comparing this polynomial with the general equation from above, we see that:
• a = 5,
,
• b = -3,
,
• c = -2.
To find the number of intersections, we compute the discriminant of this polynomial:
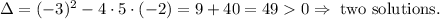
So we have two intersections.
(b) The values of x at the intersections are:
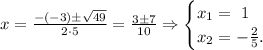
Replacing these values in the equation of one of the polynomials, we get:
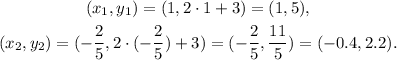
Answer
(ii)
• (a) There are 2 intersections
,
• (b) The intersections are (1,5) and (-0.4, 2.2)