We need to find the probabilities for each required z-score.
A z-score table shows the probability associated with values less than a specific z-score.
Thus, in order to use a z-score table to solve this problem, we need to notice that the probability that z is greater than a certain number equals the whole probability (1) minus the probability that z is less than that number.
The probability associated with a z-score less than 2.25 is shown above.
We have the table below with some negative z-scores:
Thus, we have:
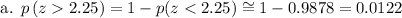

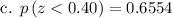
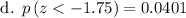
Answers
a. 0.0122
b. 0.8849
c. 0.6554
d. 0.0401