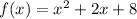Question

The amount of energy a microwave oven uses (in the form of electricity) is given by the function f(x)=5x+9, where x is the amount of time the microwave is turned on. The amount of energy the microwave puts out is modeled by the function g(x)=−x2+3x+1, where x is the amount of time the microwave is turned on.What is the difference between the amount of energy the microwave uses and the amount it puts out?

Answer 1

Given the functions:

`\begin{gathered} f(x)=5x+9 \\ \\ g(x)=-x^2+3x+1 \end{gathered}`

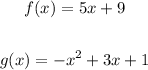
Where f(x) is the amount of energy the microwave uses and g(x) is the amount of energy it puts out.

Let's find the difference between the amount of energy the microwave uses and the amount it puts out.

To find this difference, we have:

`\begin{gathered} f(x)-g(x) \\ \\ =(5x+9)-(-x^2+3x+1) \end{gathered}`

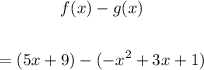
Apply distributive property and remove the parentheses:

`\begin{gathered} 5x+9+x^2-3x-1 \\ \\ \end{gathered}`

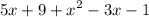
Combine like terms and reorder the expressions:

`\begin{gathered} x^2+5x-3x+9-1 \\ \\ x^2+2x+8 \end{gathered}`

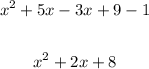
Therefore, the difference between the amount of energy the microwave uses and the amount it puts out is:

`f(x)=x^2+2x+8`

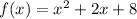
ANSWER:

`f(x)=x^2+2x+8`