Hello,
The formula for finding the area of a circular region is:
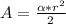
then:
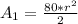
With the two radius it is formed an isosceles triangle, so, we must obtain its area, but first we obtain the height and the base.
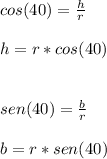
Now we can find its area:
![A_(2)=2* (b*h)/(2) \\ \\ A_(2)= [r*sen(40)][r*cos(40)]\\ \\A_(2)= r^(2)*sen(40)*cos(40)](https://img.qammunity.org/2015/formulas/mathematics/high-school/iswed6t2xh0g4v7rvaaqcgrmk2vinggjzx.png)
The subtraction of the two areas is 100cm^2, then:
 Answer: r= 1.59cm
Answer: r= 1.59cm