We have to calculate the number of data within 2 population standard deviation of the mean.
For we have to find the mean of the given data.
Mean is given by
![\operatorname{mean}=(87+63+39+67+66+63+62+67+66)/(9)=(580)/(9)=64.44]()
The standard deviation is given by the formula,
![\sigma=\sqrt[]{\frac{\Sigma(x_i-\operatorname{mean})^2}{9}}]()
Then the standard deviation is given by
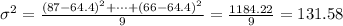
Hence the standard deviation is
![\sigma=\sqrt[]{131.58}=11.47](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/qg8502zayhc47ii5614h.png)