Question

write an equation in slope-intercept form for the line passing through each pair of points. (1/2,1) and (1,5)

Answer 1

The equation of our line is y = 8x - 3

Step-by-step explanation

The slope-intercept form of writing the equation of the straight line is given as

y = mx + c

where

y = y-coordinate of any point on the line

m = slope of the line

x = x-coordinate of the point on the line whose y-coordinate is y.

c = y-intercept of the line; the value of y when x = 0.

To write this equation, we first compute the slope of the line

The slope of a line with two points on the line with the coordinates (x₁, y₁) and (x₂, y₂) given is calculated as

`\text{Slope = }\frac{Change\text{ in y}}{Change\text{ in x}}=(y_(2)-y_(1))/(x_(2)-x_(1))`

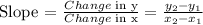
For this question,

(x₁, y₁) and (x₂, y₂) is (1/2, 1) and (1, 5)

y₂ = 5

y₁ = 1

x₂ = 1

x₁ = (1/2) = 0.5

`\text{Slope = }(5-1)/(1-0.5)=(4)/(0.5)=8`

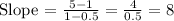
So, the slope of this line = 8

The equation of the line is then

y = 8x + c

We can now solve for the y-intercept by taking any of the two points given

Using (1, 5), x = 1, y = 5

y = 8x + c

5 = 8(1) + c

5 = 8 + c

c = 5 - 8 = -3

Hence, the equation of our line is y = 8x - 3

Hope this Helps!!!