Question

A pyramid with a square base has a volume of 144 m. Its height is twice its width. What is its height?

Answer 1

Square base pyramid

We know that the volume of a square base pyramid is 144m³. And we know we can find it using the following equation:

`\text{vol}=(1)/(3)base\text{ area}\cdot h`

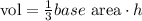
where h: height.

Since the base is a square, the area of the base is given by its width

area = w · w = w²

then, replacing in the first equation:

`\text{vol}=(1)/(3)w^2h=144m`

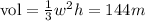
where w: width.

On the other hand, we have that its height, h, is twice its width, w:

2w = h

↓

w = h/2

Then, replacing w by h in our equation:

`\begin{gathered} \text{vol}=(1)/(3)((h)/(2))^2\cdot h=144m^3 \\ \\ \\ \\ \\ \\ \end{gathered}`

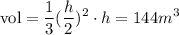
since the exponent is distributed to every part of the fraction we have:

`\begin{gathered} \\ \\ ((h)/(2))^2=(h^2)/(2^2)=(h^2)/(4) \\ \downarrow \\ (1)/(3)((h)/(2))^2\cdot h=(1)/(3)\cdot(h^2)/(4)^{}\cdot h \\ \\ \end{gathered}`

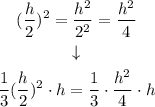
in order to operate them we can conver h into a fraction:

`\begin{gathered} \\ \\ h=(h)/(1) \\ \downarrow \\ (1)/(3)\cdot(h^2)/(4)^{}\cdot h=(1)/(3)\cdot(h^2)/(4)^{}\cdot(h)/(1)=(1\cdot h^2\cdot h)/(3\cdot4\cdot1)^{}=(h^3)/(12) \\ \downarrow \\ (h^3)/(12)=144m^3 \end{gathered}`

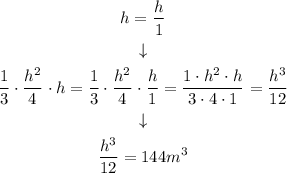
Now, we can solve the equation for h:

`\begin{gathered} \frac{h^3}{12^{}}=144m^3 \\ \downarrow \\ h^3=144m^3\cdot12=1728m^3 \\ \downarrow \\ h=\sqrt[3]{1728m^3} \end{gathered}`

We have that

`\sqrt[3]{1728m^3}=12m`

Then, h = 12 m (and w = 6m)

Answer: its height is 12m