Question

Figure 1 illustrate a circuit consists of series and parallel resistors. By using Ohm’s Law, determine following parameters:i) total resistance of the circuit, Reqii) current iiii) voltage Vxiv) power dissipated at R5

Answer 1

i) Proceed as follow:

Sum R6, R7 and R8 in parallel:

`\begin{gathered} (1)/(R^(\prime))=(1)/(R6)+(1)/(R7)+(1)/(R8) \\ (1)/(R^(\prime))=(1)/(2000\Omega)+(1)/(550\Omega)+(1)/(1000\Omega) \\ R^(\prime)\approx301.37\Omega \end{gathered}`

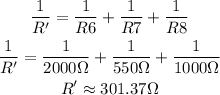
Next, sum R3 and R4 in parallel;

`\begin{gathered} (1)/(R^(\doubleprime))=(1)/(R3)+(1)/(R4) \\ (1)/(R^(\doubleprime))=(1)/(900\Omega)+(1)/(1500\Omega) \\ R^(\doubleprime)=562.5\Omega \end{gathered}`

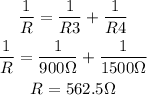
Then, you obtain a circuit with only resistances in series. Sum R1, R2, R'', R5, R' and R9 in series:

`\begin{gathered} R_{\text{tot}}=R1+R2+R^(\doubleprime)+R5+R^(\prime)+R9 \\ R_{\text{tot}}=550\Omega+1000\Omega+562.5\Omega+220\Omega+301.37\Omega+200\Omega \\ R_{\text{tot}}=2833.87\Omega \end{gathered}`

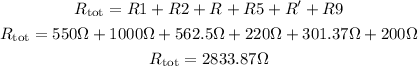
The previous result is the total resistance of the circuit

ii) Current I is given by:

`i=\frac{V}{R_{\text{tot}}}=(80V)/(2833.87\Omega)=0.028A`

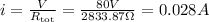
The current is 0.028A

iii) Voltage at Vx is:

`V_x=i\cdot R5=(0.028A)(220\Omega)=6.16V`

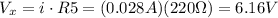
The voltage is 6.16V

iv) The power dissipated at R5 is:

`P=i^2R5=(0.028A)^2(220\Omega)=0.17W`

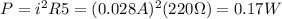
Hence, the power dissiapted at R5 is approximately 0.17W