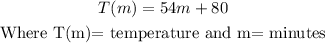The desired temperature is 350 degrees Fahrenheit at 5 minutes.
The oven's temperature is 134 degrees Fahrenheit at 1 minute.
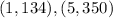
Since, it is a linear function, we have;

Then, applying the point slope formula, we have;
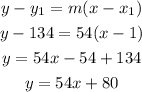
Relating it back to the context of the question, we have;