Question

How many ways can first, second, and third place be awarded to 3 contestants?

Answer 1

For use to be able to determine how many ways can first, second, and third place be awarded to 3 contestants, we will be using the permutation formula.

`\text{ nPr = }\frac{n!}{(n\text{ - r)!}}`

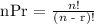
Where,

P = the number of permutations

n = total number of objects in the set = 3 contestants = 3

r = number of choosing objects from the set = 1st, 2nd & 3rd place = 3

We get,

`\text{ nPr = }\frac{n!}{(n\text{ - r)!}}`
`\text{ }_3P_3\text{ = }(3!)/((3-3)!)`
`\text{= }\frac{3\text{!}}{0!}\text{ = }\frac{\text{ 3 x 2 x 1}}{1}`
`\text{ = }\frac{\text{6}}{1}\text{ = 6}`

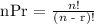
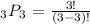
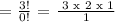
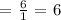
Therefore, there are 6 possible ways can first, second and third place be awarded to 3 contestants.

The answer is 6.