Given:
A certain disease has an incidence rate 0.6%. If the false negative rate is 4% and the false positive rate is 4%.
To know:
Probability that a person who tests positive actually has the disease.
Step-by-step explanation:
We can use Bayes theorem to calculate.
Solution:
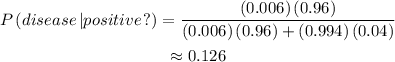
Hence 0.126 is the required probability.