If we want to find the area of the shaded region, we would have to find the area of the circle and the area of the triangles, and subtract the last result from the area of the big shape.
Area of the circle
Using the formula for the area of the circle, we have:
![\begin{gathered} A=\pi\cdot r^2 \\ A=\pi(10\operatorname{cm})^2\text{ (Replacing the values)} \\ A=\pi(100\operatorname{cm})(\text{ Raising 10 to the power of 2)} \\ A=314\text{ cm}^2 \end{gathered}]()
Area of the triangles:
Angle θ is equal to 360° divided by 8 given that we have 8 equal parts. Angle θ =45.
We are going to find the area of each triangle with the formula A=(1/2)(a)(b)(sin(C). In this case a=10 cm ,b=10 cm and sin(C)=sin(45)=0.707.
Replacing in the formula, we have:
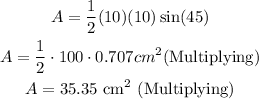
The area of the 8 triangles is: 35.35 x 8 cm^2 = 282.8 cm^2 (Multiplying)
Area of the shaded region
Area of the circle - Area of the triangles = 314 cm^2 - 282.8 cm^2 = 31.2 cm^2
The answer is 31.2 cm^2 rounded to three significant figures.