Answer:
After 15 years, there will be 3.150 grams of substance.
Explanation:
Remember that the general exponential function for decay is:
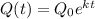
Where Q(t) is the quantity after t years, and Qo is the initial quantity.
Since we know that there were 10 grams initially and that after 9 years only 5 grams remain, we can say that:

Solving for k,
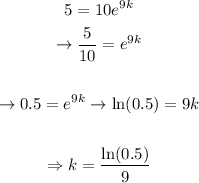
This way, we'll have that:
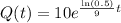
We can calculate how much substance is left after 15 years as following:
![\begin{gathered} Q(15)=10e^{\frac{\operatorname{\ln}(0.5)}{9}*15} \\ \\ \rightarrow Q(15)=3.150 \end{gathered}]()
We can conclude that after 15 years, there will be 3.150 grams of substance.