Question

Hello. thanks for viewing my question. im totally stuck on this

Answer 1

Step-by-step explanation

We can find the area of the rectangle and then subtract the area of the semicircles to find the area of the paper that remains. Both semicircles form a circle. Then, we have:

`A=A1-A2`

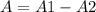
Finding the area of the rectangle

The formula to find the area of a rectangle is:

`A_{\text{ rectangle}}=\text{ length }\cdot\text{ width}`

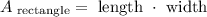
Then, we have:

`\begin{gathered} A1=34cm*16cm \\ A1=544cm^2 \end{gathered}`

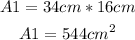
Finding the area of the circle

The formula to find the area of a circle is:

`\begin{gathered} A_{\text{ circle}}=\pi r^2 \\ \text{ Where} \\ \text{r is the radius of the circle} \end{gathered}`

The radius is half of the diameter. Then, we have:

`\begin{gathered} \text{ radius }=\frac{\text{ diameter}}{2} \\ \text{rad}\imaginaryI\text{us}=(16cm)/(2) \\ \text{rad}\imaginaryI\text{us}=8cm \end{gathered}`
`\begin{gathered} A2=\pi r^2 \\ A2=\pi(8cm)^2 \\ A2=64\pi cm^2 \end{gathered}`

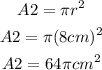
Calculating the area of the paper that remains

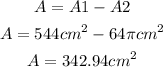
`\begin{gathered} A=A1-A2 \\ A=544cm^2-64\pi cm^2 \\ A=342.94cm^2 \end{gathered}`Answer

The area of the paper that remains rounding the nearest hundredth is 342.94 cm².