Question

Find the answer to this at least 3 decimal places

Answer 1

A.) Measure of Angle B,

The sum of all interior angles of a triangle is equal to 180 degrees.

As shown in the photo, it appears that the triangle is a right triangle, thus, one of its interior angles measures 90 degrees aside from Angle C that measures 55 degrees.

Thus, Angle B must be,

`\text{ }\angle A\text{ + }\angle B\text{ + }\angle C=180^(\circ)`
`\text{ }90^(\circ)\text{ + }\angle B\text{ + }55^(\circ)=180^(\circ)`
`\angle B\text{ + }145^(\circ)=180^(\circ)`
`\angle B\text{ }=180^(\circ)\text{ - }145^(\circ)`
`\angle B\text{ }=35^(\circ)`

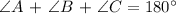
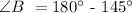
Therefore, the measure of Angle B is 35 degrees.

B.) The length of AB.

For us to be able to get the length of AB, we will be using the Tangent Function.

We get,

`\text{ Tangent (}\theta)\text{ = }\frac{\text{ Opposite Side}}{\text{ Adjacent Side}}`
`Tangent(35^(\circ))\text{ = }\frac{\text{ 1}3}{\text{ AB}}`
`AB\text{ = }\frac{\text{ 1}3}{Tangent(35^(\circ))}`
`\text{ AB = }18.565924087647488528088040304981\text{ }\approx18.566`

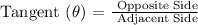
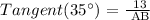
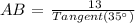
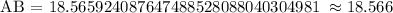
Therefore, the length of AB is 18.566

C.) The length of BC.

For us to be able to determine the length of BC, we will be using the Sine Function.

We get,

`\text{ Sine (}\theta)\text{ = }\frac{\text{ Opposite Side}}{\text{ Hypotenuse}}`
`\text{ Sine (}35^(\circ))\text{ = }\frac{\text{ 1}3}{\text{ BC}}`
`\text{ BC = }\frac{\text{ 1}3}{\text{ Sine (}35^(\circ))}`
`\text{ BC = }22.664808343074274257889884417417\text{ }\approx\text{ 22.665}`

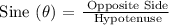
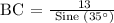
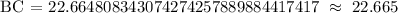
Therefore, the length of BC is 22.665