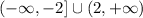Question

Solve the compound inequality.– 2 x >4or4x + 1>9Write the solution in interval notation.If there is no solution, enter Ø.

Answer 1

This is a compound inequality. We need to take a look first if the two inequalities will be a union of sets or an intersection of sets. Since the problem used or for the two inequalities, the solution set will be a union of sets.

Let's now solve for the values of x for the given inequalities. We have

`\begin{gathered} -2x\ge4 \\ x\leq-2 \end{gathered}`
`\begin{gathered} 4x+1>9 \\ 4x>8 \\ x>2 \end{gathered}`

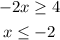
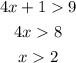
Using interval notation, the first inequality shows a solution set wherein the accepted values of x start from negative infinity up to -2. Since the first inequality has less than or equal to the symbol, the bracket symbol must be used to close the set. The second inequality shows a solution set that starts from 2 to positive infinity. Hence, the union of solution sets for these two solutions can be written as

`(-\infty,-2\rbrack\cup(2,+\infty)`