Question

Hello! I’m trying to figure out this problem but have no idea how.

Answer 1

Given: Two investments compounded annually as follows-

`\begin{gathered} P_1=4800 \\ R_1=7\% \end{gathered}`

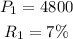
and,

`\begin{gathered} P_2=7900 \\ R_2=5.7\% \end{gathered}`

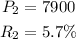
Required: To find out the time required by smaller investment to catch up to larger investment.

Explanation: The formula for compound interest is as follows-

`A=P(1+(r)/(100))^t`

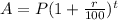
Putting the values for the first investment (smaller one) we get,

`\begin{gathered} A_1=4800(1+(7)/(100))^t \\ =4800(1+0.07)^t \\ =4800(1.07)^t \end{gathered}`

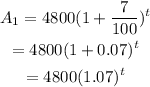
Similarly solving for other investment we get,

`\begin{gathered} A_2=7900(1+0.057)^t \\ =7900(1.057)^t \end{gathered}`

Now lets say in time 't' our investment catch up or becomes equal, i.e.,

`A_1=A_2`
`4800(1.07)^t=7900(1.057)^t`
`((7900)/(4800))=((1.057)/(1.07))^t`
`1.65=(0.98)^t`

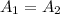
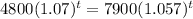
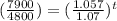
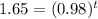
Taking logarithm both sides and solving for t gives us,

`t\approx40.7599`
`\approx40.8`

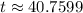
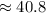
Final Answer: The investments would catch up after approximately 40.8 yrs.