Question

A guide wire is 35 feet long and is used to support the top of a tent pole, which is 21 feet tall. What angle does the wire make with the ground? What angle does it make with the top of the pole?What process would I use to solve this equation?

Answer 1

Here we have to use the trigonometric formula for right-angled triangle.

This is the structure described in the question. We have to find x which is the angle between the wire and the ground.

From the trigonometric formula of right angled triangle we know that

`\sin x=\frac{opposite\text{ side}}{\text{hypotenuse}}`

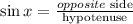
So

`\sin x=(21)/(35)\Rightarrow\sin x=(3)/(5)\Rightarrow x=\sin ^(-1)((3)/(5))\Rightarrow x=36.8642`

At the top of the pole the wire will make angle with the pole is

`\cos y=\frac{Adjacent}{\text{Hypotenuse}}\Rightarrow\cos y=(21)/(35)\Rightarrow y=\cos ^(-1)((3)/(5))\Rightarrow y=53.1301`

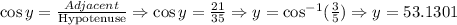
So the wire makes a 53.1301-degree angles with the top of the pole.