Question

I need help to find the answer to this problem

Answer 1

Therefore, the area of the pool cover = 380.285 feet square

while the length of the rope equals 69.14 feet.

Step-by-step explanation:

From the question, the pool has a diameter of 20 feet and the cover extends 12 inches

we need to convert 12 inches to feet

so 12 inches is equivalent to 1feet

Since the pool is circular, we need to use the formula for finding the area of a circle

Area of circle =

`\pi r^2`

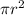
Now, the radius of the pool equals half of the diameter of the pool

The radius of the pool =

`(1)/(2)*20=10`

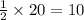
Also, the radius of the cover will be = radius of the pool + 12 inches(converted to 1 feet)

hence, the radius of the cover =

`10+1=11`

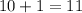
Now, using the formula for the area of circle=

`\begin{gathered} \pi r^2 \\ =(22)/(7)*11^2=((22*121))/(7) \\ =380.285ft^2 \\ ^{} \\ \\ \end{gathered}`

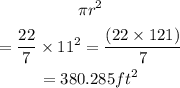
Therefore the area of the pool cover = 380.285 feet square

Also, the length of the rope would be the perimeter of the pool cover which is calculated using the formula for finding the perimeter of the circle

which is:

`=2\pi r`

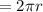
Hence the lenght of the rope =

`\begin{gathered} =2\pi r \\ =2*(22)/(7)*11 \\ =69.14ft \end{gathered}`

Therefore, the area of the pool cover = 380.285 feet square

while the lenght of the rope equals 69.14 feet.