Question

the magnitude of an earthquake is measured on the Richter scale as a logarithm of the intensity of the shock wave. for magnitude R and intensity I, the formula is R=log(I). the June 28, 1992 earthquake in landers measured 7.3 on the Richter scale. the oceanside earthquake on September 20, 2001 measured 3.1 on the scale. how many times more intense was the landers earthquake than the oceanside earthquake? round your answer to two decimal places, if necessary.

Answer 1

In order to calculate how many times one earthquake is more intense than the other, first let's calculate the intensity of each one.

To do so, let's use the definition of logarithm:

`R=\log _(10)(I)\to10^R=I`

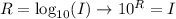
So we have:

`\begin{gathered} \text{landers: R = 7.3} \\ 7.3=\log (I_1) \\ 10^(7.3)=I_1 \\ \\ \text{oceanside: R = 3.1} \\ 3.1=\log (I_2) \\ 10^(3.1)=I_2 \end{gathered}`

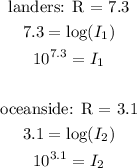
Now, to calculate the division of intensities, we can use the property below:

`(a^b)/(a^c)=a^(b-c)`

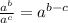
So we have:

`(I_1)/(I_2)=(10^(7.3))/(10^(3.1))=10^(7.3-3.1)=10^(4.2)=15848.93`

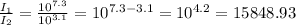
Therefore the intensity of the first earthquake was 15848.93 times higher than the intensity of the second earthquake.