Question

4 Ellie is in a snowboarding competition. The function (t) =- 16t² + 30t + 10 models Ellie's height, & in the air afterseconds. Use the quadratic formula to determine, to the nearest tenth of a second, at what two times her height was 15 feet.Show all steps

Answer 1

Given: Ellie's height function

`\begin{gathered} H(t)=-16t^2+30t+10 \\ H(t)=Height \\ t=time \end{gathered}`

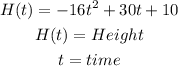
To Determine: The two times when the height was 15 feet

Solution

Given the quadratic formula below

`\begin{gathered} A\text{ quadratic equation below} \\ ax^2+bx+c=0 \\ Using\text{ quadratic formula} \\ x=(-b\pm√(b^2-4ac))/(2a) \end{gathered}`

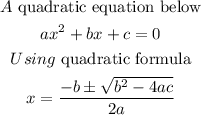
When height is 15 feet

`\begin{gathered} 15=-16t^2+30t+10 \\ 16t^2-30t-10+15=0 \\ 16t^2-30t+5=0 \end{gathered}`

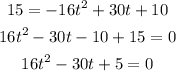
Using quadratic formula

`\begin{gathered} a=16,b=-30,c=5 \\ t=(-(-30)\pm√((-30)^2-4*16*5))/(2*16) \end{gathered}`
`t=(-\left(-30\right)\pm\:2√(145))/(2\cdot\:16)`
`t_1=(-\left(-30\right)+2√(145))/(2\cdot \:16),\:t_2=(-\left(-30\right)-2√(145))/(2\cdot \:16)`
`\begin{gathered} t_1=(30+24.083)/(32),t_2=(30-24.083)/(32) \\ t_1=(54.083)/(32),t_2=(6.083)/(32) \end{gathered}`
`\begin{gathered} t_1=1.69,t_2=0.19 \\ t_1\approx1.7seconds,t_2=0.2seconds \end{gathered}`

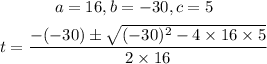
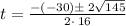
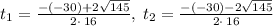
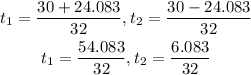
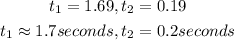
Hence, the two times Ellies height was 25 feet is 1.7 seconds and 0.2 seconds