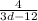Question

Using v=lwh, what is an expression for the volume of the following prism?

Answer 1

Option C is correct

`(4)/(3d-12)`

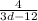
Explanation:

Using the formula:

`V = lwh`

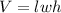
where,

l is the length of the prism

w is the width of the prism

h is the height of the prism.

As per the statement:

From the given figure:

Length of the prism(l) =
`(d-2)/(3d-9)`

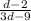
Width of the prism(w) =
`(4)/(d-4)`

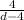
Height of the prism(w) =
`(2d-6)/(2d-4)`

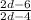
Substitute these in [1] we have;

`V = (d-2)/(3d-9) \cdot (4)/(d-4) \cdot (2d-6)/(2d-4)`

`V = (d-2)/(3(d-3)) \cdot (4)/(d-4) \cdot (2(d-3))/(2(d-2))`

⇒
`V = (1)/(3) \cdot (4)/(d-4) \cdot (2)/(2)`

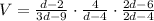
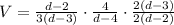
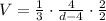
Simplify:

⇒
`V = (4)/(3(d-4))`

⇒
`V = (4)/(3d-12)`

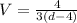
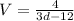
Therefore, the expression for the volume of the following prism is,
`(4)/(3d-12)`

Answer 2

we know that

The volume of the prism is equal to

`V=L*W*H`

where

L is the length side of the base of the prism

W is the width side of the base of the prism

H is the height of the prism

In this problem we have

`L=(d-2)/(3d-9)=(d-2)/(3(d-3))`

`W=(4)/(d-4)`

`H=(2d-6)/(2d-4)=(2(d-3))/(2(d-2))=((d-3))/((d-2))`

Substitute the values in the formula

`V=(d-2)/(3(d-3))*(4)/(d-4)*((d-3))/((d-2))=(4)/(3(d-4))=(4)/(3d-12)`

therefore

the answer is the option

`(4)/(3d-12)`