Question

Suppose you had a chance to work for 22 weeks and could choose one of two methods of payment. You could choose to be paid $1 the first week, $2 the second week, $4 the third week, $8 the fourth week, etc., with the amount doubling each week; or you could choose to receive $2 million in one lump sum. Which method would result in the greater payment? Explain.

Answer 1

Given:

The first method of payment follows a sequence:

1, 2, 4, 8,...

The second method of payment is a lump sum of $2 million

The final amount for the first method can be found using the formula:

`\begin{gathered} S_n=\text{ }(a(r^n-1))/(r-1) \\ Where\text{ r is the common ratio} \\ and\text{ a is the first term} \end{gathered}`

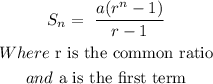
a = 1

r = 2

n =22

Substituting the values:

`\begin{gathered} S_n\text{ = }\frac{1(2^(22)\text{ -1\rparen}}{2-1} \\ =\text{ 4194303} \end{gathered}`

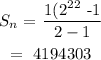
The final amount is $4194303

Hence, the first method would result in the greater payment because it would yield $4 million while the second method would yield $2 million