Question

May I need help with this. I have tried multiple times to get the correct or at least accurate answers but still could was all incorrect I’d appreciate it so much if had help

Answer 1

We have to find the measures of the angles.

The diagonal bisects the angles of the rhombus, so ∠2 should have the same measure as 41°, the angle on the other side of the diagonal.

Consecutive angles from the rombhus will be supplementary: their measures will add 180°.

Then, if one of the angles is 2*41° = 82°, the consecutive, like ∠3, will be:

`\begin{gathered} m\angle3+82\degree=180\degree \\ m\angle3=180-82=98\degree \end{gathered}`

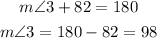
Angle ∠1 is opposite to ∠3, and following the rule that consecutive angles are supplementary, they should have the same measure, as they are supplementary to the same angles.

Angle ∠4 and ∠2 are alternate interior angles between parallels, so they will have the same measure (41°).

NOTE: we can check that the angles in each triangle will add 180°, as 41+41+98 = 180°.

Answer:

m∠1 = 98°

m∠2 = 41°

m∠3 = 98°

m∠4 = 41°